
International Journal of Optics and Photonic Engineering
(ISSN: 2631-5092)
Volume 7, Issue 2
Research Article
DOI: 10.35840/2631-5092/4550
Structural Dynamics of Light Propagation
Richard Sauerheber, PhD*
Table of Content
- Abstract
- Introduction
- Methods
- Results and Discussion
- Doppler Shifts in Frequency and Wavelength
- Light Speed Measurements Mathematically Justified
- Synchronous Aberration
- Observed and Theoretic Light Speed
- Error in Estimating Time Using Light Clocks
- Light and the Standard Meter
- Intrinsic Structure of the Photon
- Supply file
Figures

Figure 1: Melting bands (indicated by vertical...
Melting bands (indicated by vertical lines) in chocolate substrate reveal the observed wavelength of microwave radiation. Vertical bands of melted chocolate are separated at an average distance of 2.65 cm in a microwave resonant cavity designed with a 2450 Hz magnetron. The resonant waves energize and induce vibration in molecules in the substrate which generates heat of melting. Temperature first rises at locations of EM radiation electric field maxima and minima. This separation distance is thus a half wavelength of the emitted radiation. The observed wavelength is twice this distance, at 0.127 m and may be used to estimate the intrinsic speed (but not the relative velocity) of the EM radiation.
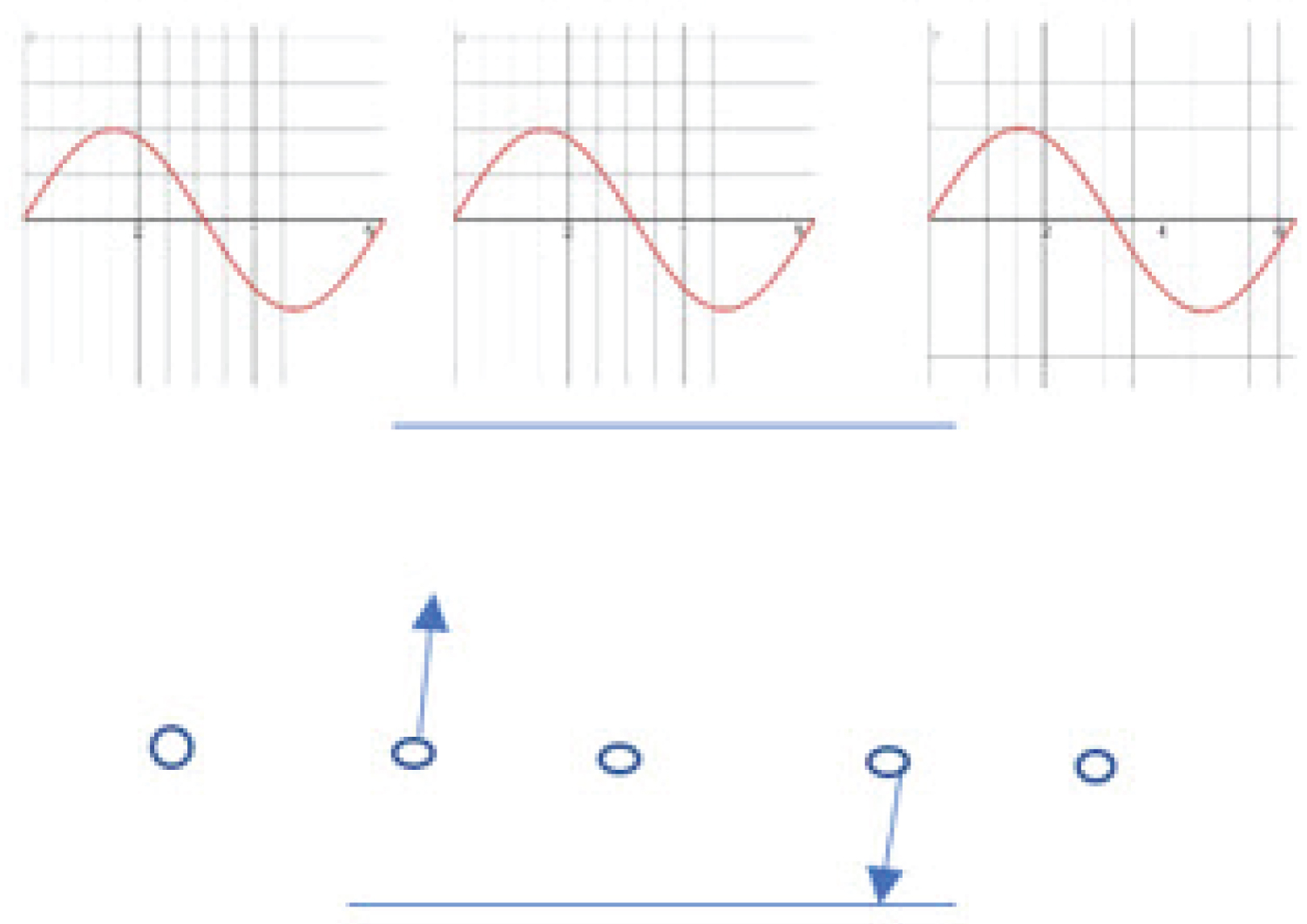
Figure 2: Representations of a single....
Representations of a single photon traveling through space are drawn according to two distinct viewpoints. If a photon were an intact wavelet, the wave itself simply travels forward, propagating energy along an entire pathline across any affected matter (upper figures showing presence of a wave at three different positions of increasing time from left to right). However, if photons are corpuscles of energy that contain electric and magnetic fields that are not of constant amplitude but oscillate, tracing out a sinusoidal wave pattern in space through which the photon travels, then the electric and magnetic field amplitudes would also oscillate along its pathline that would have maxima, minima, and nodes at distinct locations along a substrate. The lower circles represent photon corpuscles having a maximum and minimum E and B field (with amplitude represented by lengths of arrows), and three nodal positions as time increases from left to right. Although the maximum amplitudes of the fields can be computed for any particular frequency [2], the distance over which the fields emanate into space is not known and are represented here only for purpose of discussion.
References
- Giancola D (2009) Physics for scientists and engineers. Prentice Hall, New Jersey.
- Sauerheber RD (2021) Intrinsic Properties of the Photon. Int J Opt Photonic Eng 6: 045.
- https://en.wikipedia.org/wiki/Speed_of_light
- Arthur O (1960) Light velocity and relativity. Burckel and Associates, Yonkers-on-Hudson, NY.
- Hertz H (1893) Electric waves: Being researches on the propagation of electric action with finite velocity through space. Dover Publications.
- Sauerheber RD, Espinoza E (2018) Characteristics of light: Velocity, massless energy, and special relativity. Optik 168: 974-986.
- Einstein A (1923) Electrodynamics of bodies in motion 1905. In: The Principle of Relativity, Methuen and Co. Ltd., London.
Author Details
Richard Sauerheber, PhD*
Palomar Community College, 1140 W. Mission, San Marcos, CA 92069, USA
Corresponding author
Richard Sauerheber, Ph.D., Palomar Community College, 1140 W. Mission, San Marcos, CA 92069, USA.
Accepted: October 20, 2022 | Published Online: October 22, 2022
Citation: Sauerheber R (2022) Structural Dynamics of Light Propagation. Int J Opt Photonic Eng 7:050.
Copyright: © 2022 Sauerheber R. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.
Abstract
An analysis of the properties of light produced by sources in motion helps elucidate the mechanism of light transmission through space. Photons, as massless corpuscles of electromagnetic energy, trace out wave patterns of electric and magnetic field amplitude while propagating forward at rapid speed. Coherent sources of electromagnetic energy thus can cause heating of material in a particular pattern depending on the frequency of the light. Such melting band patterns are measured here on physical substrates to deduce the nature of light transmission in the microwave frequency range. Doppler effects on frequency and wavelength, but not intrinsic light speed, for sources in motion are compensated when detectors share the same velocity. Therefore, light intrinsic speed computed from the frequency and wavelength of the light are accurate when source and detector have no relative velocity between them. The distance a photon travels after being generated by a moving source for a single oscillation wave cycle is not the observed wavelength that would exist in the absence of motion. These findings help understanding photon structure and light propagation through space which might improve the efficiency of various optical systems.
Introduction
Light is electromagnetic energy given by E = hf where h is Planck's constant and f is the frequency of light [1]. This energy contains no term for mass because light has no mass. So EM energy intrinsic to light is radically different than the intrinsic energy that physical objects with mass have, given by E = mc2. Light photons have electric and magnetic field components, of equal energy at any instant, that trace wave patterns through space while propagating forward. But the structure of photons themselves are debated, being either intact waves or corpuscles that trace wave patterns. Although the dimensions of a single photon packet of energy are not accurately known, this has been addressed in an earlier study [2].
Generated waves that propagate though space are subject to Doppler effects when sources have motion, which alter the frequency and wavelength of the waves as a function of the velocity of the source. All light sources in the known universe undergo motion. Light from orbiting twin stars is Doppler shifted regularly. Light from the receding side of the spinning sun is red shifted while light emanating from the approaching side of the sun is blue shifted. Light from man-made electrical devices on earth also have a velocity equal to the velocity of the earth. We here examine the properties of microwave EM energy in a resonant cavity of known calibrated frequency [3] that are produced and then detected while in motion on the orbiting earth, to characterize features of light propagation through space.
Methods
Microwaves were generated in a Sunbeam Model SGKJ701 microwave oven manufactured in Shindo, China. The unit was calibrated initially at 2450 MHz on a 60 Hz cycle 110 V circuit. The cavity contained no rotating base, to enable measurement of the wavelength of the microwaves. This was done by measuring the distance between hot melted bands on various substrates. The substrates used were layered gelatin and flat bars of chocolate. Since microwave frequencies energize molecules in the substrate to vibrate and generate melting heat first at positions of electrical amplitude minima and maxima, the separation distance between melted bands corresponds to one half wavelength of the radiation.
Results and Discussion
Light waves having a frequency of 2450.0 Hz from a calibrated magnetron generator produce radiation with an altered frequency when the source is in motion, due to Doppler shifting effects on wavelength and frequency. During summertime when the earth orbits the sun-earth common barycenter at approximately 66,000 mph (29.498 km/s), ignoring additional velocity of lesser magnitude due to the angular rotation of the galaxy, and any residual velocity due to possible translational motion of the universe of matter, the actual frequency of this radiation may be estimated for instructional purposes using well established Doppler shift formula.
Doppler Shifts in Frequency and Wavelength
The produced frequency f' would be increased by the motion of the earth when the radiation propagates in the direction the earth orbits and would be decreased when the radiation propagates in the direction opposite the orbital velocity of the earth. When the source and earth velocity is in the same direction of wave propagation, the produced frequency would be:
f ' = f/(1 - vs/c) [1] where vs is the velocity of the earth and source as a positive value, c is the speed of light under the particular conditions, and f is the electronically generated intrinsic frequency of the magnetron that generates the radiation. Here f ' = 2450.2 MHz, assuming c = 2.9971 × 108 m/s, the accepted value for 50% humidity air at sea level pressure and room temperature. When oriented so that the direction of light propagation is opposite earth orbit velocity, the produced frequency would be f ' = 2449.8 MHz where vs is a negative quantity.
The produced wavelength λ' associated with this frequency of light may be computed from c = f 'λ', where λ' = 0.12235 m for the direction the earth orbits and 0.12237 m for the opposite direction. This wavelength differs from the intrinsic wavelength λ that would be generated in the absence of any known motion of the source, at λ = c/f = 0.12236 m.
Predicted produced frequencies for orientations skewed for the direction of earth orbit travel are more complex, where light photons have the lateral velocity of a lateral moving source while angle traveling to detectors at speed c [2] and are outside the scope of this discussion.
Light Speed Measurements Mathematically Justified
Light evidently travels through space generating wave tracings due to EM oscillations in time. Since the speed of light is fixed at c from the location in space at which light is produced and this speed is independent of the motional velocity of the source, this means that the distance a photon travels while generating a wave tracing is not equal to the wavelength that would be generated by the stationary source of the radiation. If a source were perfectly stationary, which is not possible in the known universe of motional matter, the distance of light travel during one wavelength oscillation would exactly equal the wavelength generated by the source frequency. But light sources move, so actual light propagation frequencies and wavelengths are different than when sources are stationary. Since the speed of light remains constant c regardless of source motion, the distance traveled by a photon while tracing a single oscillation is dependent on the velocity of the source and never exactly equals the original wavelength that would be generated by a source that is stationary.
Measurements of the wavelength observed on physical matter heated in a microwave source enable the computation of the intrinsic (but not relative) speed of light from a moving source/detector system. The relative velocity between a light front and a moving detector of course are unknown because the actual total velocity of the earth is not known. If the source used here could be made to remain stationary, again the frequency and wavelength would be f = 2450.0 MHz and λ = 0.12236 m. If the source and detector move together at the same velocity vs, then there is no relative velocity between them, and the observed wavelength should be expected to be this same value. Theoretically this can be explained by the fact that the Doppler shift in wavelength caused by the moving earth, where the source moves toward the detector, is exactly compensated by the shifted wavelength that would occur due to motion of the detector away from the source. For the system used here:
λobs = c/fobs = c/[f '(1 - vs/c)] = 0.12236 m.
This observed wavelength is indeed the wavelength that would have been produced for a 2450.0 Hz source if it could be made stationary. Although the light propagates through space with a higher frequency and shorter wavelength than this due to the Doppler shift of the source in the direction of light propagation, the observed wavelength (and frequency) are corrected automatically by the motion of the detector away from the source having the same velocity. In other words, propagating light from all moving sources has a different wavelength than if the sources were stationary, but the observed wavelength is the same as that from the stationary, non-Doppler-shifted, source when there is no relative velocity between source and detector. This adds to the detailed description by Otis [4] of the altered light frequency and wavelength that are detected due to relative motion of detectors.
Synchronous Aberration
This result also is consistent with an earlier description of the phenomenon termed synchronous aberration [2]. Light most commonly observed, that is light sources on earth such as light bulbs or other electronic devices and objects that reflect sunlight, undergo synchronous aberration where images of objects that are sensed by the eye were actually reflected from the object at an earlier time. But since both the object and observer are in coincident motion, the object remains behind the image at all times while the light angle travels to the observer. Similarly, the observed wavelength and frequency observed in the present experiments are able to be used to compute correctly the intrinsic speed that light has even though the actual frequency and wavelength for the propagating radiation are Doppler-shifted. As the magnitude of c remains fixed regardless of the state of motion of the source, the Doppler changes in frequency and wavelength due to motion of the earth source are compensated by the coincident motion of the detector so that intrinsic light speed can be measured on the moving earth. The early computations of light speed as a multiple of wavelength and frequency by Hertz [5] are fully valid even though the source of the radiation and the detector were both in motion on earth at the time. Note that the propagating frequency and wavelength of light cannot actually be accurately known since all sources have unknown total velocities and Doppler shifts.
Observed and Theoretic Light Speed
Microwave radiation melt lines on a substrate were here used to estimate the wavelength of EM radiation of a known calibrated frequency. The discovery that led to the development of microwave ovens to heat food occurred when it was accidentally noted that a chocolate bar melted during experiments with microwaves [3]. Here we used both gelatin and chocolate as substrates to measure observed wavelength.
Since the substrates used here are moving with the velocity of the earth, as is the source of the radiation, the true frequency and true wavelength of the radiation propagating through space differs from values if the system could be made stationary. However, there is no relative velocity between the substrate and source, so the observed wavelength should match that which would have been observed if the source and substrate were stationary. The measured wavelengths were computed from the distance between melt bands multiplied by 2, where the melt bands first occur at positions of maximum and minimum amplitude, over a distance of one-half wavelength. From λ = c/f, the theoretically expected wavelength at light speed 2.9979 × 108 m/s is estimated at 0.12236 m to five-digit precision.
The observed wavelength directly measured with a metric instrument from the melted gelatin substrate, accurate to three digits, is 0.127 m (2 × 0.0635 cm observed). Using chocolate, the average wavelength observed was 0.133 m (2 × 0.067 m). The error in measuring the separation distance between melting regions is about 0.1 cm which is a 1.5% error.
The measured intrinsic speeds for light computed here from c = fλ were 0.127 m × 2450 × 106 Hz = 3.1 × 108 m/s and 0.133 m × 2450 × 106 Hz = 3.25 × 108 m/s respectively. The difference between observed light speed (and wavelength) versus expected theoretic is about 6.6%. This difference may be due to several factors. The speed of light in moist air differs from that in dry air (at 2.9979 × 108 m/s) but the percentage difference is only 0.03%, at 2.9971 × 108 m/s for a 50% average humidity atmosphere at sea level pressure. Although 110 V voltage can vary by about 10%, these variations occurring during a 15 second measurement here are much smaller. Frequency variations from 60 Hz used by Southern California power companies are regulated to vary by no more than ± 2.5%. Finally, the difference in frequency and voltage supplied to the unit in Southern CA and Shindo, China are considered significant. Taken together it appears that the explained error mostly arises from both the deviation from the original calibrated frequency for the magnetron that generates the microwaves used here and the measurement error for the melting positions on the substrates, together an error of approximately 4%.
It is clear that the intrinsic speed (but not the relative velocity) of light may be correctly computed from such measurements of frequency and wavelength in systems that have a relatively rapid velocity such as the orbiting, spinning earth in the solar system with its significant angular velocity. This is consistent with the known fact that the speed of light is a value intrinsic to light itself, independent of motion of its source or detector, or Doppler effects on frequency. The intrinsic speed of light is fixed at c for all bands of the EM spectrum, from highest frequency gamma rays to lowest frequency radio waves [1,6] which may be expressed at a massive 11.2 million miles per hour or 18 million kilometers per hour.
However, the relative velocity of a light beam or its components of course depend on the velocity of the point of reference, where for a detector receding from a wave front at speed v, light relative velocity would be c - v and for an approaching detector would be c + v [7] as pointed out originally by Einstein in 1905. The simplest explanation for the known constant speed of light derived initially by James Clerk Maxwell in 1864 as c = E/B = 1/(eu)0.5, and later confirmed by Hertz in 1887 as c = fλ is that c is the intrinsic speed for light with respect to the location in space at which it is produced. E and B are the magnitudes of the orthogonal electric and magnetic fields forming the radiation. Speed magnitudes are always relative to a particular position, and light speed being always a constant refers to its speed with respect to a stationary point in space or to the spatial coordinate in space at which it leaves its source. Elegant determinations of f and λ to high accuracy made by Hertz are not possible with our simple microwave cavity but are not necessary for the intended purpose here.
The relative velocity of a light front with respect to a moving source is also determined by the velocity of the source, but the velocity of earth-bound sources is not actually known, due to all the motions, known and unknown, that the earth undergoes. So total velocities of physical entities cannot actually be known. However, since planet velocities are small compared to light speed, relative velocities for light differ from intrinsic light speed by only small percentages. Although miniscule electrons have been energized to near light speed, objects with large mass such as galaxies should not reach velocities of such magnitude.
Error in Estimating Time Using Light Clocks
Unfortunately, the time of propagation of light computed from a system such as used here, a 'light clock', would be sensitive to motion. This is because the actual time traveled for one oscillation wave from the moving source would be 1/f ~ 0.41 ns. During this time the detector also moves while the speed of light remains fixed. This means that the presumed or observed distance along the detector that the light passes while the detector also moves is different than the distance the light actually travels in space during the oscillation. Computing time from the observed distance on the physical detector divided by c is invalid, since c is the intrinsic velocity of light, not the relative velocity with respect to the moving system. If one used the actual Doppler shifted wavelength λ' divided by c, the time would be correct, but this is not known to an observer who uses the detector to estimate the distance light travels along it as though that were the actual wavelength propagated in space. Here the relative distance light travels along the moving detector substrate during the time the radiation completes one oscillation is d = λ' + vt where v is the velocity of the detector and t is the time for light to imprint one oscillation. But since there is no relative velocity between source and detector d = λ, the wavelength that would have been produced if there had been no source/detector motion or Doppler shift. The observed wavelength λ is longer than the wavelength λ' light has while propagating in space and equals the wavelength the stationary source would have produced.
It is therefore not accurate to compute time with a light box, where physical objects in motion are used to detect it. Estimates of time can be made only by also considering the relative motion between light source and detector, such as a star and the earth, or a light clock and the location in space at which the light departs the source in the light clock. For example, the time required for light to travel the observed distance 0.12235 m is λ/c = 0.4081 ns. This is not the true time theoretically computed from of λ'/c = 0.4082 ns. This is because the time required to travel one Doppler shifted wavelength λ' is indeed the value t = λ'/c. It is not the observed doubled distance between burn spots d = λ divided by speed c because this distance moved by the detector is longer than the actual single wavelength of propagation λ' for the Doppler shifted radiation (here assumed for the case where light propagates in the earth orbit direction). This further extends earlier observations that the idea of time dilation due to motion is a computation error or a measurement error, rather than being the actual slowing of absolute time [6].
Light and the Standard Meter
Finally, since the wavelength is altered by motion, a different number of wave oscillations would be required to reach a target detector than if the source were stationary. Source motion toward a target causes a shorter wavelength in that direction, and in the case where the detector is in motion away from the light front, with no relative velocity between source and detector, this further increases the number of oscillation necessary to arrive at the target. The reverse is true if the source recedes from the target and the target approaches the light front. These effects complicate the method of standardizing the length of a meter based on a particular number of wavelengths of travel from a source with a given frequency (Figure 1).
Intrinsic Structure of the Photon
The experiments here help to determine the true structural nature of individual photons. It is widely held that a photon is a single wave or wavelet, where the energy in a light sample is given by E = nhc/λ so that at n = 1 photon, this seems to compute the energy of a single wave of light. However, it is also possible that an individual photon, made by a single electronic energy transition that emits it, is a miniscule corpuscle of energy that oscillates and thus traces out a wave pattern behind it while propagating forward. Here the computed energy of a single photon represents the energy the photon contains as an oscillating corpuscle generating a wave pattern, rather than being an intact wave itself. If a photon transports its energy through space as a pre-formed intact individual wavelet, then the electric and magnetic field maxima would uniformly pass-through a given region of space, causing an equal effect on a substrate along its length. But if the photon is instead a miniscule corpuscle of energy that oscillates its electric and magnetic field amplitudes, tracing out a wave pattern in space, than the field maxima and minima would only exist at specific antinode locations that the photon passes on the substrate. Nodal positions where the electric and magnetic fields have amplitudes of zero would also occur at other locations on the substrate that the photons pass.
The present experiments suggest that photons indeed trace out a wave pattern in space and are not intact wavelets themselves. Specific locations at which field amplitudes are maximal cause heating and melting of the substrates used here, while at other regions between these locations the field amplitudes are of lesser amplitude or zero. If a photon were a pre-formed wavelet, then the entire substrate would be melted uniformly as the radiation passes, even though ironically oscillations in field amplitudes would still occur while passing any and all particular locations along the substrate. The data clearly indicate separated melting lines form with a wavelength consistent with known light speed c. This indicates that photons are packets of energy that have maximum field amplitudes at specific locations in space rather than across a spatial region. Thus, photons are not traveling intact waves, but instead appear to be packets of electromagnetic energy that trace out wave patterns behind them while propagating forward at speed c. These distinct possibilities for the structure of photons are shown in Figure 2.
The resonant cavity length is approximately 12 inches (30.5 cm) which would constrain by reflection about 2.5 resonant wave patterns, or about five half wavelengths within it while being continuously irradiated. Because of motion of the earth-based system, the actual number of waves is slightly different, causing small errors in the distance between melting bands depending on the orientation of the system with respect to the directional velocity of the earth at the time of measurement. Since light speed is massive compared to earth total velocity, the difference of course is not easily detected with the methods employed here, estimated on the order of a few microns. The fact that half waves of photon propagation establish a resonant condition causing the melting bands indicates that photons are not formed intact entities with a size equaling their wavelength.
A third possibility, that a photon could consist of an intact half-wavelet that oscillates within itself, so that peaks in field amplitude would occur at regularly spaced intervals while propagating forward, requires that there is a time when the entire wavelength would have a field amplitude of zero. Microwaves travel forming a 2 inch wavelength here, and radiowaves trace out a wave pattern of 10 million meters length (at 3 Hz), larger than the radius of the entire earth, so this is not considered tenable. Long entities would not follow the law of reflection where angles of incidence and reflection must be equal. These distinctions are important since it is widely argued that a photon is actually an intact wave.
The notion that all photons are miniscule in size may explain the similarities in behavior of all EM radiation, from low energy photons tracing out radio waves near 5 × 106 m in length, to high energy gamma radiation with miniscule wavelengths near 10-12 m. Photons are neither particles with mass nor waves but of course have properties of particles, as in the photoelectric effect or Compton scattering, and properties of waves in being reflected, diffracted, and refracted without change in speed. It must be emphasized that light is unique in the world of Physics by having mas-like properties but no mass, electric fields but no net charge, and magnetic fields but no net magnetic dipole.
Earlier evidence suggested that photons might compare in size to that of an electron, estimated at 10-15 meters [2]. Indeed a photon must be no larger than its own wavelength, and an electron diameter is less than the wavelength of gamma rays. The magnitude of the electric field maximum for each photon of frequency 2450 × 106 Hz in vacuum would be E = (hf/εo)0.5 = (6.63 × 10-34 J/s)(2450 × 106 Hz)/(8.85 × 10-12 C2/N-m2)0.5 = 6.06 × 10-7 V/m. The energy for each photon would be E = hf = 1.63 × 10-24 J/photon. The 700 Watt energy of the system produced a maximum of (700 J/s)/(1.63 × 10-24 J/photon) = 4.29 × 1026 photons each second in the melting experiment. The combined action of all produced photons causes the heating of substrates. Electrons oscillating along a wire or antenna each shift only a small distance during an oscillation to generate radio waves where each produce a photon each cycle. The number of photons per cycle produced here would require an estimated 1.75 × 1017 (4.29 × 1026 photons/s)(1 s/2450 × 106 cycles) electrons producing photons each oscillation.
Light is well-known to have characteristics that particles with mass have, and also to have characteristics that waves have. But light is technically neither a physical particle nor an intact wave. Particles have physical mass, but light has no mass. Light exhibits diffraction and Doppler shifts due to motion of sources, as waves so exhibit, but photons appear to trace wave patterns behind them, rather than being propagating intact waves themselves. Source/detector systems with no relative velocity measure intrinsic wavelengths of light even while light propagates between source and detector at Doppler-shifted frequencies (Supplemental notes).

